Tourist level has still the same color as LGM in the chart: 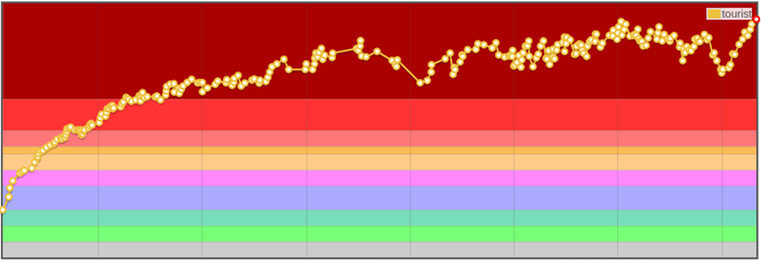
| № | Пользователь | Рейтинг |
|---|---|---|
| 1 | tourist | 3985 |
| 2 | jiangly | 3814 |
| 3 | jqdai0815 | 3682 |
| 4 | Benq | 3529 |
| 5 | orzdevinwang | 3526 |
| 6 | ksun48 | 3517 |
| 7 | Radewoosh | 3410 |
| 8 | hos.lyric | 3399 |
| 9 | ecnerwala | 3392 |
| 9 | Um_nik | 3392 |
| Страны | Города | Организации | Всё → |
| № | Пользователь | Вклад |
|---|---|---|
| 1 | cry | 169 |
| 2 | maomao90 | 162 |
| 2 | Um_nik | 162 |
| 4 | atcoder_official | 160 |
| 5 | djm03178 | 158 |
| 6 | -is-this-fft- | 157 |
| 7 | adamant | 155 |
| 8 | Dominater069 | 154 |
| 8 | awoo | 154 |
| 10 | luogu_official | 151 |
You can make a mashup contest of problems you want to solve and send your solution in a group. Your code will be judged in a few minutes!

I tried a greedy solution for this problem:
1. If there exist a pair of adjacent vertices like $$$(u,v)$$$ such that $$$deg(u)\geq3$$$ and $$$deg(v)\geq3$$$, then remove the edge between $$$u$$$ and $$$v$$$.
2. Then, if there exist a pair of adjacent vertices like $$$(u,v)$$$ such that $$$deg(u)\geq3$$$ and $$$deg(v)\geq2$$$, then remove the edge between $$$u$$$ and $$$v$$$.
3. Then, if there exist a pair of adjacent vertices like $$$(u,v)$$$ such that $$$deg(u)\geq3$$$ and $$$deg(v)\geq1$$$, then remove the edge between $$$u$$$ and $$$v$$$.
At last, I add edges between the leaves of different components .
Why isn't it correct??
submission
Given a graph G s.t. any cycle in G has length 3.
1)Find the maximum number of edges. 2)Find the maximum number of cycles.
Can someone compare atcoder contests and problem levels with codeforces contest and problem levels?
| Название |
|---|










