Chasty's blog

Help me
By Chasty, history, 9 years ago, In English

I am learning DP and I am a litle frustrated. I am stucked in this problem http://codeforces.me/problemset/problem/466/C I drew some pictures to find out some states this is the image http://i61.tinypic.com/de4rpe.jpg theres is a pattern in these pictures to get this formula n = n — 2 numberOfTotalCombinations = n * (n-1) / 2 the worst case(5 * 10^5) would be = 124999750000 and it will not pass. My questions Should I consider all the combinations? How to define my states? How I need to construct a DP solution based on the previous states? Thansk in advance and sorry for my bad english

  • Vote: I like it
  • 0
  • Vote: I do not like it

Comments Comments (2)
| Write comment?
»
9 years ago, # |
← Rev. 4   Vote: I like it 0 Vote: I do not like it

No, you shouldn't consider all combinations. This is DP, it's all about avoiding cases where you can't get a result. This problem is solved this way: Let: x=sum(k=1, i-1)a[k]; y=sum(k=i, j)a[k]; z=sum(k=j+1, n)a[k]; and s=sum(k=1, n)a[k], the sum of all numbers in the array We observe that x+y+z=s, and how x=y=z (that's what the problem states) => 3*x=3*y=3*c=z, thus x=y=z=s/3. You can figure it out from here, but here is the rest of the solution (dont't read unless you gave up on the problem): Let v be an array such as v[i]=sum(k=1, i)a[k]. If vn is not divisible by 3, that means we can't divide s into three equal parts. Now, we will work with the sums in the array v. So, how x=y=z=s/3, we need to find all sums equal with s/3. The number of ways we can achieve that sum is the number of x's we can obtain. Afterwards, when we can no longer obtain s/3, we try to obtain 2*s/3 (from array v), that is x+y. When we find one, we add the number of ways of getting x to the solution. The code looks something like this: http://codeforces.me/contest/466/submission/11713930

→ Reply
»
9 years ago, # |
  Vote: I like it +1 Vote: I do not like it

If you consider all possible combinations, then it's not DP, but brute froce. Anyway, this problem is something else.

Let S be the sum of all elements. First of all, if that sum is not a multiple of 3, then the answer is 0. OK, now calculate Li, which means how many positions p are there with p ≤ i such that the sum of all elements from 1 to p is equal to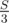 . Then iterate from the right (from N down to 1) and keep track of the sum of all elements so far. If at position i, that sum is 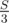, add Li - 2 to the answer (you must have at least one element in the middle section).

→ Reply