Hi —
Recently I took look at problem from Hackerrank BACK TO SCHOOL CODESPRINT called Xor Subsequence. I'm wondering if there is solution to this problem that doesn't utilize FFT.
| # | User | Rating |
|---|---|---|
| 1 | tourist | 3857 |
| 2 | jiangly | 3747 |
| 3 | orzdevinwang | 3706 |
| 4 | jqdai0815 | 3682 |
| 5 | ksun48 | 3591 |
| 6 | gamegame | 3477 |
| 7 | Benq | 3468 |
| 8 | Radewoosh | 3463 |
| 9 | ecnerwala | 3451 |
| 10 | heuristica | 3431 |
| # | User | Contrib. |
|---|---|---|
| 1 | cry | 167 |
| 2 | -is-this-fft- | 162 |
| 3 | Dominater069 | 160 |
| 4 | Um_nik | 158 |
| 4 | atcoder_official | 158 |
| 6 | Qingyu | 156 |
| 7 | djm03178 | 151 |
| 7 | adamant | 151 |
| 7 | luogu_official | 151 |
| 10 | awoo | 146 |
Hi —
Recently I took look at problem from Hackerrank BACK TO SCHOOL CODESPRINT called Xor Subsequence. I'm wondering if there is solution to this problem that doesn't utilize FFT.
| Name |
|---|



Frankly saying I don't know an answer to your question, but I will leave this as a fun fact that if we will allow also empty subsequences of form [i, i) (so in fact we increase number of occurences of zero by n) then answer will be always 0 :). It follows from an inequality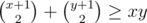 which shows up in comparing number of occurences of zeros and some other value. Without adding those empty intervals that inequlity changes to
which shows up in comparing number of occurences of zeros and some other value. Without adding those empty intervals that inequlity changes to 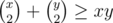 , which in fact is not true :(. Or maybe I should say ":)", because that means that this problem has any sense :D.
, which in fact is not true :(. Or maybe I should say ":)", because that means that this problem has any sense :D.