→ Pay attention
→ Top rated
| # | User | Rating |
|---|---|---|
| 1 | Benq | 3676 |
| 2 | Kevin114514 | 3659 |
| 3 | jiangly | 3640 |
| 4 | turmax | 3450 |
| 5 | tourist | 3408 |
| 6 | strapple | 3360 |
| 7 | Um_nik | 3354 |
| 8 | squareOf105 | 3348 |
| 9 | maroonrk | 3312 |
| 10 | idontwannadothis…e | 3191 |
→ Top contributors
| # | User | Contrib. |
|---|---|---|
| 1 | Qingyu | 154 |
| 2 | nik_exists | 151 |
| 3 | maspy | 149 |
| 4 | AmShZ | 146 |
| 5 | Um_nik | 143 |
| 6 | Errichto | 139 |
| 7 | BledDest | 136 |
| 8 | adamant | 135 |
| 9 | maroonrk | 133 |
| 10 | cry | 130 |
→ Find user
→ Recent actions
Codeforces (c) Copyright 2010-2026 Mike Mirzayanov
The only programming contests Web 2.0 platform
Server time: Nov/09/2026 20:00:30 (h1).
Desktop version, switch to mobile version.
Supported by
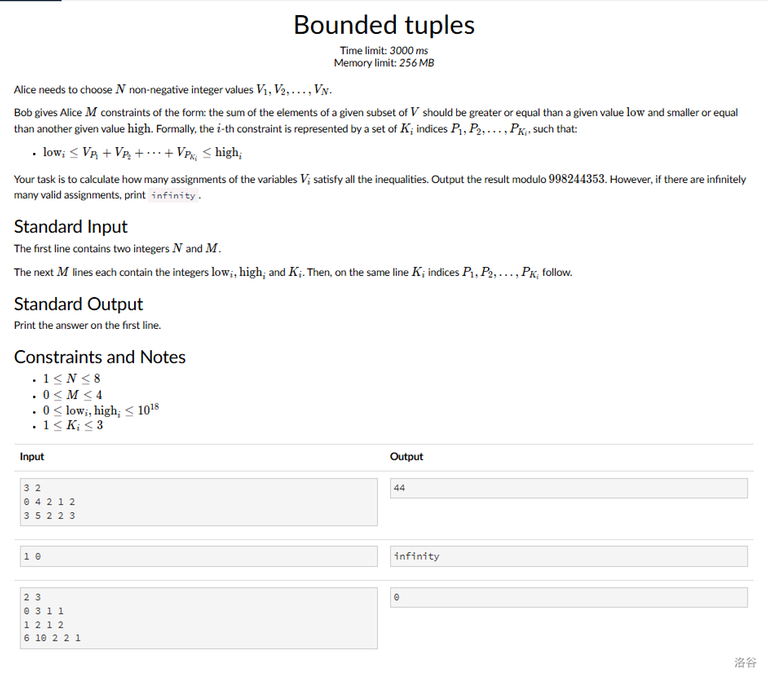
It seems like a very constrained version of the "count integer points inside a polytope" problem. For fixed dimension there are polynomial solutions for it. See: A Polynomial Time Algorithm for Counting Integral Points in Polyhedra When the Dimension Is Fixed [AI Barvinok · 1994].
I don't know for sure, but possibly you can maybe simplify this paper a bit and just implement that. Still that seems really hard. I don't know if there's any easier ideas.
Thx, I’ll give the paper a read. appreciate your help here!