You are given a hexagon in which all his angles are equal in measure, you draw a circle inscribed inside the hexagon and tangent to the hexagon's sides, and another circle which is the circumscribed circle of the hexagon (all the hexagon vertices lie on the circumference of this circle), suppose the side length of the hexagon is $$$X$$$, find the area of the ring between the two circles in terms of $$$X$$$.
The ring in yellow is the required ring
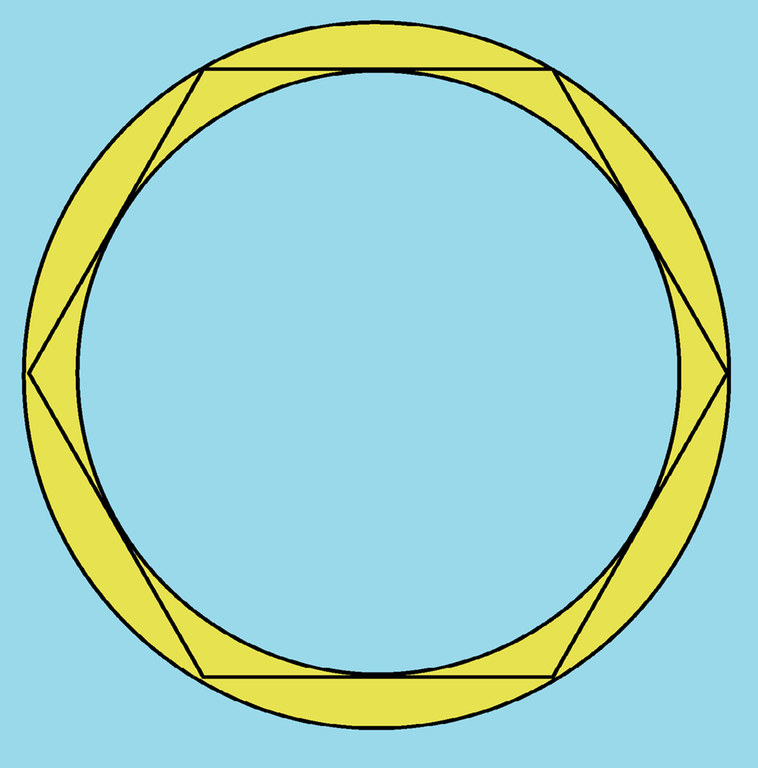
BONUS : Find a general solution in terms of $$$N$$$ and $$$X$$$ (where polygon $$$P$$$ is a regular $$$N$$$th-gon of side length $$$X$$$ each) for the area of the ring between the circle inscribed in $$$P$$$ and the circle $$$P$$$ is inscribed in











Use a normal font size like everyone else. It is like screaming. (It is rude.)
I thought it would fit for this relatively large photo, but Ok
Option 2: make the photo smaller?
Makes more sense, but I made that photo in the past and i was too lazy to edit it XD
https://en.wikipedia.org/wiki/Regular_polygon#Circumradius
This should be all that you need. And the formula for the area of a circle on the radius.
I mean I dont have a lot of trigonometry stuff , but I learned vectors here and there and I think since we know the side length of the polygon and each sides degree with the x axis , we can just do some vector addition like
1st vector is , length n , degree is 270 — 60
2nd vector is ,length n , degree is 60
1st vector + 2nd vector's = sqrt( (x1+x2)^2 + (y1 + y2)^2) that is the radius of the smaller circle
u can do the same with 3 vectors for finding the bigger circles radius I hope Im correct please fix me If Im wrong